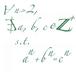1. FC's Axiom
A. ma ∀X∀Y∀a∀b (X ma a ∧ Y ma b ∧ X=Y) ⇒ a=b
A. el ∀X∀Y (∀b b∈X ⇔ b∈Y) ⇒ X=Y
A.F ∃x∃B (∀a a ∈ B ⇔ F(a)) ∧ B ma x
Where X, Y, Z, .... are the variable for Atsumari , a, b, c, .... are the variable for Class
A. ma represents that the Atsumari makes only one class
A. el represents that the Atsumari is decided by its member
A.F is Axiom schema of unrestricted comprehension
A. F represents if you collect Classes which have property F then it becomes an Atsumari B and the Atsumari B makes a Class x
2. Translation to FC
The translation of ZF's formula to FC's formula is the following
x ∈ y in ZF is translated to ∃ B x ∈ B ∧ B ma y in FC - TR –
3. Russel's class
∀a a∈R ⇔ ¬(∃B a∈B ∧ B ma a)
The right side of formula means ¬(a∈a) in ZF Using TR
This Atsumari R makes Russel's Class r, so R ma r.
Let a=r then,
r∈R ⇔ ¬(∃B r∈B ∧ B ma r)
If r∈R is true, considering that R ma r is also true, then
∃B r∈B ∧ B ma r is true, then right side of formula is false. This is a contradiction.
So, the following formulas are gotten.
¬(r∈R), ∃B r∈B ∧ B ma r.
Idea “Set “ :
I use the word "Set" for representing a scale of a Class.
The definition of Set is the following.
Set x Df. ¬(∃B x∈B ∧ B ma x)
Then Russel's Class is written as follows.
∀a a∈R ⇔ Set a
And results are,
¬(r∈R), ¬Set r
4. Ordinal Number
Ordinal Number is defined by Ordinal Atsumari that is transitive and well - ordering in the same way in ZF
Ord x Df. ∃ B Ord B ∧ B ma x
Ord B Df. Transitive B ⋀ Well – Ordering B
Transitive A Df. ∀x x∈A ⇒ ∃!B B<A ∧ B ma x
Well - Ordering A Df. ( ∀x∀y x∈A ∧ y∈A ⇒ x<y | A V y<x | A V x=y ) ⋀ ( ∀B (∃b b∈B) ∧ B⊂A ⇒ ∃a a∈B ∧ (∀b b<a|A ⇒ ¬b∈B) )
Where x<y | A Df. ∃B (x∈B ∧ B ma y) ∧ B⊂A
B⊂A Df. (∀a (a∈B ⇒ a∈A)) ∧ ¬(A=B)
B⊂A means B is a proper sub-Atsumari of A.
5. Model
On FC , Finite Model exists M5 is defined as follows:
U={0,1,2,3, n}
{ } ma 0, {0} ma 1, {0,1} ma 2, {0,1,2} ma 3, {0,1,2,3} ma n, {0,1,2,3,n} ma n,
For all other Atsumari X, X ma n
It is possible to verify the result of section 3. " Russel's Class " on M5.
On M5, classes 0, 1, 2, 3 are Set.
So, R={0,1,2,3}
and r=n , because {0,1,2,3} ma n
The two formulas of a conclusion of the section 3. are ¬(r∈R) , ∃B r∈B ∧ B ma r.
Both are true , because ¬(n∈{0,1,2,3}) and n∈{0,1,2,3,n} ∧ {0,1,2,3,n} ma n
Any model of FC satisfies all axioms of ZF except the axiom of foundation.
In FC, a class x such that ∃B x∈B ∧ B ma x exists, which means x∈x in ZF.
So, the model of FC doesn't satisfy the Axiom of Foundation
On M5, P(U)=U, naturally, 1-1 function between U and U exists. The existence of 1-1 function between P(U) and U is not contradiction
Where P(U) means part Atsumari of U
U Df. ∀x x∈U
Notice on M5 Fermat's Theorem is incorrect
2^3 + 3^3 = 3^3 = n
Where "-" and "+" are defined in the same way in ZF
In M5 , Ord U So , Ordinal Number is represented
But it is possible to prove M5 is consistent , because M5 is finite
A. ma ∀X∀Y∀a∀b (X ma a ∧ Y ma b ∧ X=Y) ⇒ a=b
A. el ∀X∀Y (∀b b∈X ⇔ b∈Y) ⇒ X=Y
A.F ∃x∃B (∀a a ∈ B ⇔ F(a)) ∧ B ma x
Where X, Y, Z, .... are the variable for Atsumari , a, b, c, .... are the variable for Class
A. ma represents that the Atsumari makes only one class
A. el represents that the Atsumari is decided by its member
A.F is Axiom schema of unrestricted comprehension
A. F represents if you collect Classes which have property F then it becomes an Atsumari B and the Atsumari B makes a Class x
2. Translation to FC
The translation of ZF's formula to FC's formula is the following
x ∈ y in ZF is translated to ∃ B x ∈ B ∧ B ma y in FC - TR –
3. Russel's class
∀a a∈R ⇔ ¬(∃B a∈B ∧ B ma a)
The right side of formula means ¬(a∈a) in ZF Using TR
This Atsumari R makes Russel's Class r, so R ma r.
Let a=r then,
r∈R ⇔ ¬(∃B r∈B ∧ B ma r)
If r∈R is true, considering that R ma r is also true, then
∃B r∈B ∧ B ma r is true, then right side of formula is false. This is a contradiction.
So, the following formulas are gotten.
¬(r∈R), ∃B r∈B ∧ B ma r.
Idea “Set “ :
I use the word "Set" for representing a scale of a Class.
The definition of Set is the following.
Set x Df. ¬(∃B x∈B ∧ B ma x)
Then Russel's Class is written as follows.
∀a a∈R ⇔ Set a
And results are,
¬(r∈R), ¬Set r
4. Ordinal Number
Ordinal Number is defined by Ordinal Atsumari that is transitive and well - ordering in the same way in ZF
Ord x Df. ∃ B Ord B ∧ B ma x
Ord B Df. Transitive B ⋀ Well – Ordering B
Transitive A Df. ∀x x∈A ⇒ ∃!B B<A ∧ B ma x
Well - Ordering A Df. ( ∀x∀y x∈A ∧ y∈A ⇒ x<y | A V y<x | A V x=y ) ⋀ ( ∀B (∃b b∈B) ∧ B⊂A ⇒ ∃a a∈B ∧ (∀b b<a|A ⇒ ¬b∈B) )
Where x<y | A Df. ∃B (x∈B ∧ B ma y) ∧ B⊂A
B⊂A Df. (∀a (a∈B ⇒ a∈A)) ∧ ¬(A=B)
B⊂A means B is a proper sub-Atsumari of A.
5. Model
On FC , Finite Model exists M5 is defined as follows:
U={0,1,2,3, n}
{ } ma 0, {0} ma 1, {0,1} ma 2, {0,1,2} ma 3, {0,1,2,3} ma n, {0,1,2,3,n} ma n,
For all other Atsumari X, X ma n
It is possible to verify the result of section 3. " Russel's Class " on M5.
On M5, classes 0, 1, 2, 3 are Set.
So, R={0,1,2,3}
and r=n , because {0,1,2,3} ma n
The two formulas of a conclusion of the section 3. are ¬(r∈R) , ∃B r∈B ∧ B ma r.
Both are true , because ¬(n∈{0,1,2,3}) and n∈{0,1,2,3,n} ∧ {0,1,2,3,n} ma n
Any model of FC satisfies all axioms of ZF except the axiom of foundation.
In FC, a class x such that ∃B x∈B ∧ B ma x exists, which means x∈x in ZF.
So, the model of FC doesn't satisfy the Axiom of Foundation
On M5, P(U)=U, naturally, 1-1 function between U and U exists. The existence of 1-1 function between P(U) and U is not contradiction
Where P(U) means part Atsumari of U
U Df. ∀x x∈U
Notice on M5 Fermat's Theorem is incorrect
2^3 + 3^3 = 3^3 = n
Where "-" and "+" are defined in the same way in ZF
In M5 , Ord U So , Ordinal Number is represented
But it is possible to prove M5 is consistent , because M5 is finite
|
|
|
|
|
|
|
|
「自由クラス理論」非標準集合論 更新情報
-
最新のイベント
-
まだ何もありません
-
-
最新のアンケート
-
まだ何もありません
-
「自由クラス理論」非標準集合論のメンバーはこんなコミュニティにも参加しています
人気コミュニティランキング
- 1位
- パニック障害とうつ病
- 8448人
- 2位
- 一行で笑わせろ!
- 82529人
- 3位
- 写真を撮るのが好き
- 208285人