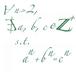3. Theorem
Theorem for the properties which Class Theory of each type has.
[ Theorem of Type.1 ]
T.1−1 ¬Obj u
T.1−2 Set u [A.Cl]
T.1−3 ∀b Prcl b ⇒ Set b
T.1−4 ∃k Ind k
Individual is able to exist.
A.Pair We ashume this Axiom
Set u Hence
{u} ma k So
Set k
Class k is Set, so it is possible to assume k is Obj
Obj k −*−
From T.1−1
¬Obj u Hence
¬At {u} Hence
¬Cl k [A.Cl]
From *
Ind k
Where Class k which Atsumari {u} whose member is Universal class u
makes is able to be Individual
[ A.*** ] means the Axiom *** is asumed
T.1−5 ∀y Set_ob
In Class Theory of this type, Ind and Obcl and Prcl are all Set.
For example, Class r which is ¬Set has no relationship with it
Example of Class Theory of Type.1
ZF belongs to this type.
A.Reg arrives at the condition of Type.1
[ Theorem oof Type.2 ]
T.2−1 ∀y ¬Set_ob y ⇒ Obj y −R_ob ⊂ U
T.2−2 ¬Set_ob r
T.2−3 ¬Set_ob –r_ob
T.2−4 ¬∃y Ind y [A.{ }]
T.2−5 ¬∃y Prcl y [A. Cl ]
Example of Class Theory of Type.2
FC belongs in this Type.
If we defined that all Classes are Obj then it becomes a representation of "FC" on FC. Naturally R⊂U
Obj y Df. y=y
In FC, all Classes are elements and all Classes are made by Atsumari so neither Indiviual nor Proper Class exist.
[ Theorem of Type.3 ]
T.3−0 Set_ob r [A.Cl]
T.3−1 ¬Set u ∧ Obj u [A.Cl]
[A.At]
[A.{ }]
T.3−2 ¬Set_ob u [A.Cl]
[A.At]
[A{ }]
T.3−3 ¬Set r_ob ∧ Set_ob r_ob [A.Cl]
[A.{ }]
T.3−4 ¬Set −r ∧ Set_ob −r [A.Cl]
[A.{ }]
T.3−5 ¬Set −u ∧ ¬Obj −u [A.Cl]
[A.At]
[A.{ }}
T.3−6 ¬Set_ob −r_ob [A.Cl]
[A.{ }]
T.3−7 ∀y Prcl y ⇒ Set y [A.Cl]
T.3−8 ∀y Ind y ⇒ Set y [A.At]
Example of Class Theory of Type.3
Quine's ML is an example of this Type.
We consider about such Atsumari N and Class n
∀b b∈N ⇔ b=m ∧ Set m , N ma n
"Set m" in the formula of N's condition is not able to be stratified so
¬Obj n
And with A.Pair , Set n
Hence
Set n ∧ ¬Obj n −*1−
And Universal Atsumari U is able be stratified so
Obj u
With A.⊂ , ¬Set u
Hence
¬Set u ∧ Obj u −*2−
And
Set 0 ∧ Obj 0 −*3−
Where φ ma 0
Formula *1∧*2∧*3 is the condition of Type.3
[ Theorem of Type.4 ]
T.4−1 ∀y ¬Set_ob y ⇒ Obj y
T.4−2 ¬Obj r [A.Cl]
T.4−3 ¬Set_ob u [A.Cl]
[A.{ }]
T.4−4 ¬Set_ob −r_ob [A.Cl]
[A.{ }]
T.4−5 ¬Set −r ∧ Set_ob −r [A.Cl]
[A.{ }]
T.4−6 ¬Set −u ∧ ¬Obj −u [A.Cl]
[A.At]
[A.{ }]
T.4−7 ¬Set r_ob ∧ ¬Obj r_ob [A.Cl]
[A.At]
[A.{ }]
T.4−8 ∀y ¬Prc y [A.Cl]
[A.{ }]
T.4−9 ∀y ¬Ind y [A.Cl]
No example of Class Theory of Type.4 exists in known Class Theories
Theorem for the properties which Class Theory of each type has.
[ Theorem of Type.1 ]
T.1−1 ¬Obj u
T.1−2 Set u [A.Cl]
T.1−3 ∀b Prcl b ⇒ Set b
T.1−4 ∃k Ind k
Individual is able to exist.
A.Pair We ashume this Axiom
Set u Hence
{u} ma k So
Set k
Class k is Set, so it is possible to assume k is Obj
Obj k −*−
From T.1−1
¬Obj u Hence
¬At {u} Hence
¬Cl k [A.Cl]
From *
Ind k
Where Class k which Atsumari {u} whose member is Universal class u
makes is able to be Individual
[ A.*** ] means the Axiom *** is asumed
T.1−5 ∀y Set_ob
In Class Theory of this type, Ind and Obcl and Prcl are all Set.
For example, Class r which is ¬Set has no relationship with it
Example of Class Theory of Type.1
ZF belongs to this type.
A.Reg arrives at the condition of Type.1
[ Theorem oof Type.2 ]
T.2−1 ∀y ¬Set_ob y ⇒ Obj y −R_ob ⊂ U
T.2−2 ¬Set_ob r
T.2−3 ¬Set_ob –r_ob
T.2−4 ¬∃y Ind y [A.{ }]
T.2−5 ¬∃y Prcl y [A. Cl ]
Example of Class Theory of Type.2
FC belongs in this Type.
If we defined that all Classes are Obj then it becomes a representation of "FC" on FC. Naturally R⊂U
Obj y Df. y=y
In FC, all Classes are elements and all Classes are made by Atsumari so neither Indiviual nor Proper Class exist.
[ Theorem of Type.3 ]
T.3−0 Set_ob r [A.Cl]
T.3−1 ¬Set u ∧ Obj u [A.Cl]
[A.At]
[A.{ }]
T.3−2 ¬Set_ob u [A.Cl]
[A.At]
[A{ }]
T.3−3 ¬Set r_ob ∧ Set_ob r_ob [A.Cl]
[A.{ }]
T.3−4 ¬Set −r ∧ Set_ob −r [A.Cl]
[A.{ }]
T.3−5 ¬Set −u ∧ ¬Obj −u [A.Cl]
[A.At]
[A.{ }}
T.3−6 ¬Set_ob −r_ob [A.Cl]
[A.{ }]
T.3−7 ∀y Prcl y ⇒ Set y [A.Cl]
T.3−8 ∀y Ind y ⇒ Set y [A.At]
Example of Class Theory of Type.3
Quine's ML is an example of this Type.
We consider about such Atsumari N and Class n
∀b b∈N ⇔ b=m ∧ Set m , N ma n
"Set m" in the formula of N's condition is not able to be stratified so
¬Obj n
And with A.Pair , Set n
Hence
Set n ∧ ¬Obj n −*1−
And Universal Atsumari U is able be stratified so
Obj u
With A.⊂ , ¬Set u
Hence
¬Set u ∧ Obj u −*2−
And
Set 0 ∧ Obj 0 −*3−
Where φ ma 0
Formula *1∧*2∧*3 is the condition of Type.3
[ Theorem of Type.4 ]
T.4−1 ∀y ¬Set_ob y ⇒ Obj y
T.4−2 ¬Obj r [A.Cl]
T.4−3 ¬Set_ob u [A.Cl]
[A.{ }]
T.4−4 ¬Set_ob −r_ob [A.Cl]
[A.{ }]
T.4−5 ¬Set −r ∧ Set_ob −r [A.Cl]
[A.{ }]
T.4−6 ¬Set −u ∧ ¬Obj −u [A.Cl]
[A.At]
[A.{ }]
T.4−7 ¬Set r_ob ∧ ¬Obj r_ob [A.Cl]
[A.At]
[A.{ }]
T.4−8 ∀y ¬Prc y [A.Cl]
[A.{ }]
T.4−9 ∀y ¬Ind y [A.Cl]
No example of Class Theory of Type.4 exists in known Class Theories
|
|
|
|
|
|
|
|
「自由クラス理論」非標準集合論 更新情報
-
最新のイベント
-
まだ何もありません
-
-
最新のアンケート
-
まだ何もありません
-
「自由クラス理論」非標準集合論のメンバーはこんなコミュニティにも参加しています
人気コミュニティランキング
- 1位
- 千葉 ロッテマリーンズ
- 37151人
- 2位
- mixi バスケ部
- 37849人
- 3位
- 楽天イーグルス
- 31945人