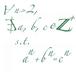11. a contradiction of { NF + some axioms }.
R and U have self-membership, so this concept has the meaning of "large".
A.1. x is large ⇒ {x} is large
A.2. b is individual ⇒ {b} is not large
Both axioms seem to be correct.
NF doesn't adjust to these two axioms.
A representation of a Set Theory on FC is defined in the next subsection “11-1. Object”
11-1. Object
If we defined "Object" in FC, then we obtain a representation of a set
theory.
Obj x Df. a formula of x
The formula in the definition describes what have sethood in the represented set theory.
ex.1. a ZF like set theory
1. Obj 0
2. Obj a ∧ Obj b ∧ {a,b} ma c ⇒ Obj c
3. One X ∧ X ma b ∧ Obj b ∧ UX ma c ⇒ Obj c
One B Df. ∀X∀Y∀c X ma c ∧ Y ma c ∧ c∈B ⇒ X=Y
UC Df. [A[b b∈UC ⇔ ∃a∃K b∈K ∧ K ma a ∧ a∈C
4. X ma b ∧ Obj b ∧ P(X) ma c ⇒ Obj c
5. A ma a ∧ B ma b ∧ A⊆B ∧ Obj b ⇒ Obj a
6. A ma a ∧ B ma b ∧ A=f"B ∧ Obj b ⇒ Obj a
f"B Df. the image of B with f.
7. 0∈X ∧ (b∈X ⇒ b'∈X) ∧ X ma c ⇒ Obj c
ex2. NF
Obj x Df. x is stratified.
11-2. object class , individual, proper class.
I define some relative ideas to Object such that "At, Cl, Obcl, Ind, Prcl."
These are abbreviations of relative "Atsumari, class, object class, individual, proper class" from a point of view on Object.
At C Df. ∀b b∈C ⇒ Obj b
If all members of an Atsu are Objects then the Atusmari is a relative Atsumari for the represented set theory.
ex.1. in NF
At {0,u} , where U ma u, ∀b∈U ⇔ b=b
Both 0 and u are stratified, so Obj 0 ∧ Obj u
ex.2. in NF
¬At {r} , where r means Russel's class.
r is not stratified, it means ¬Obj r
A.At ∀B ¬At B ⇒ (∀x x∈B ⇒ ¬Obj x)
This axiom separates Obj and ¬Obj.
Cl b Df. ∃C At C ∧ C ma b
If a relative Atsumari makes a class then the class must be a relative class for the represented set theory.
ex. in NF
Cl c , where {0,u} ma c
A.Cl ∀b Cl b ⇒ (∀X X ma b ⇒ At X)
This axiom separates At and ¬At.
Obcl b Df. Cl b ∧ Obj b
Ind x Df. ¬Cl x ∧ Obj x
Prcl c Df. Cl c ∧ ¬Obj c
These definitions are obvious.
ex. in ZF
set is object and class, so ∀b setzf b ⇒ Obcl b
where setzf means "set" in ZF.
11-3. A contradiction of NF.
We assume three axioms as follows :
A.Set. ∀C∀b (∃x x∈C ∧ C ma b ∧ ¬Set x) ⇒ ¬Set b
¬Set means something great like r or u, so if {u} ma k then k is also great, hence k must be ¬Set.
A.Obcl. ∀x∀b {x} ma b ∧ Ind x ⇒ Obcl b
For example, an apple is individual, and if {an apple} ma b then b must be Obcl.
A.Set'. ∀x∀b {x} ma b ∧ Ind x ⇒ Set' b
Set' b Df. ¬(∃C b∈C ∧ C ma b ∧ At C)
[ A proof of { NF+A.Set.+A.Set' } has a contradiction ]
Consider about Class r.
¬Set r , where r is Russel's class.
¬Set r' , where {r} ma r' . We used A,Set.
¬Set r'' , where {r'} ma r''. Again from A.Set.
And, the other hand.
¬stratified r , it is obvious.
¬Obj r , see section 1 ex,2
Ind r' , ¬At {r} and use A.Cl , so ¬Cl r'. And {r} is stratified, so Obj r'
Set' r'' , see A.Set'
So,
¬Set r'' ∧ Set' r''
It means ∃B r''∈B ∧ B ma r'' ∧ ¬At B. (F)
with A.At and the formula (F), we get ¬Obj r''.
But, stratified r'' so Obj r''. This is a contradiction.
Another conclusion ;
with A.Cl and the formula (F), we get ¬Cl r''.
But, Ind r' ∧ A.Obcl means Obcl r'', so Cl r''. It is a contradiction too.
R and U have self-membership, so this concept has the meaning of "large".
A.1. x is large ⇒ {x} is large
A.2. b is individual ⇒ {b} is not large
Both axioms seem to be correct.
NF doesn't adjust to these two axioms.
A representation of a Set Theory on FC is defined in the next subsection “11-1. Object”
11-1. Object
If we defined "Object" in FC, then we obtain a representation of a set
theory.
Obj x Df. a formula of x
The formula in the definition describes what have sethood in the represented set theory.
ex.1. a ZF like set theory
1. Obj 0
2. Obj a ∧ Obj b ∧ {a,b} ma c ⇒ Obj c
3. One X ∧ X ma b ∧ Obj b ∧ UX ma c ⇒ Obj c
One B Df. ∀X∀Y∀c X ma c ∧ Y ma c ∧ c∈B ⇒ X=Y
UC Df. [A[b b∈UC ⇔ ∃a∃K b∈K ∧ K ma a ∧ a∈C
4. X ma b ∧ Obj b ∧ P(X) ma c ⇒ Obj c
5. A ma a ∧ B ma b ∧ A⊆B ∧ Obj b ⇒ Obj a
6. A ma a ∧ B ma b ∧ A=f"B ∧ Obj b ⇒ Obj a
f"B Df. the image of B with f.
7. 0∈X ∧ (b∈X ⇒ b'∈X) ∧ X ma c ⇒ Obj c
ex2. NF
Obj x Df. x is stratified.
11-2. object class , individual, proper class.
I define some relative ideas to Object such that "At, Cl, Obcl, Ind, Prcl."
These are abbreviations of relative "Atsumari, class, object class, individual, proper class" from a point of view on Object.
At C Df. ∀b b∈C ⇒ Obj b
If all members of an Atsu are Objects then the Atusmari is a relative Atsumari for the represented set theory.
ex.1. in NF
At {0,u} , where U ma u, ∀b∈U ⇔ b=b
Both 0 and u are stratified, so Obj 0 ∧ Obj u
ex.2. in NF
¬At {r} , where r means Russel's class.
r is not stratified, it means ¬Obj r
A.At ∀B ¬At B ⇒ (∀x x∈B ⇒ ¬Obj x)
This axiom separates Obj and ¬Obj.
Cl b Df. ∃C At C ∧ C ma b
If a relative Atsumari makes a class then the class must be a relative class for the represented set theory.
ex. in NF
Cl c , where {0,u} ma c
A.Cl ∀b Cl b ⇒ (∀X X ma b ⇒ At X)
This axiom separates At and ¬At.
Obcl b Df. Cl b ∧ Obj b
Ind x Df. ¬Cl x ∧ Obj x
Prcl c Df. Cl c ∧ ¬Obj c
These definitions are obvious.
ex. in ZF
set is object and class, so ∀b setzf b ⇒ Obcl b
where setzf means "set" in ZF.
11-3. A contradiction of NF.
We assume three axioms as follows :
A.Set. ∀C∀b (∃x x∈C ∧ C ma b ∧ ¬Set x) ⇒ ¬Set b
¬Set means something great like r or u, so if {u} ma k then k is also great, hence k must be ¬Set.
A.Obcl. ∀x∀b {x} ma b ∧ Ind x ⇒ Obcl b
For example, an apple is individual, and if {an apple} ma b then b must be Obcl.
A.Set'. ∀x∀b {x} ma b ∧ Ind x ⇒ Set' b
Set' b Df. ¬(∃C b∈C ∧ C ma b ∧ At C)
[ A proof of { NF+A.Set.+A.Set' } has a contradiction ]
Consider about Class r.
¬Set r , where r is Russel's class.
¬Set r' , where {r} ma r' . We used A,Set.
¬Set r'' , where {r'} ma r''. Again from A.Set.
And, the other hand.
¬stratified r , it is obvious.
¬Obj r , see section 1 ex,2
Ind r' , ¬At {r} and use A.Cl , so ¬Cl r'. And {r} is stratified, so Obj r'
Set' r'' , see A.Set'
So,
¬Set r'' ∧ Set' r''
It means ∃B r''∈B ∧ B ma r'' ∧ ¬At B. (F)
with A.At and the formula (F), we get ¬Obj r''.
But, stratified r'' so Obj r''. This is a contradiction.
Another conclusion ;
with A.Cl and the formula (F), we get ¬Cl r''.
But, Ind r' ∧ A.Obcl means Obcl r'', so Cl r''. It is a contradiction too.
|
|
|
|
|
|
|
|
「自由クラス理論」非標準集合論 更新情報
-
最新のイベント
-
まだ何もありません
-
-
最新のアンケート
-
まだ何もありません
-
「自由クラス理論」非標準集合論のメンバーはこんなコミュニティにも参加しています
人気コミュニティランキング
- 1位
- お洒落な女の子が好き
- 90067人
- 2位
- 写真を撮るのが好き
- 208325人
- 3位
- 酒好き
- 170697人